Chapter 14 Asymptotes-II

14.1 Exercise 7

14.1.1 Question 4

Find the asymptotes of the following curves

1. x2y2−x2y−xy2+x+y+1=0$x^2{y}^2-x^{2}y-x{y}^2+x+y+1=0$

There is no x3$x^3$, so asymptotes parallel to x$x$-axis is given by equating coefficients of highest degree term of x$x$.

y2−y=0y(y−1)=0y=0,y=1$$\begin{array}{cc}{y}^2-y& =0\\ y(y-1)& =0\\ y& =0,y=1\end{array}$$

Similarly, no y3$y^3$ is present. Asymptotes parallel to y$y$-axis is given by,

x2−x=0x(x−1)=0x=0,x=1$$\begin{array}{cc}{x}^2-x& =0\\ x(x-1)& =0\\ x& =0,x=1\end{array}$$

Thus four asymptotes of the equation are,

x=0x=1y=0y=1$$\begin{array}{cc}x& =0\\ x& =1\\ y& =0\\ y& =1\end{array}$$

---

2. x2(x−y)2−a2(x2+y2)=0$x^2(x-y{)}^2-a^2(x^2+y^2)=0$

Degree of equation is 4$4$. The equation does not have asymptotes parallel to x$x$-axis. The asymptotes parallel to y$y$-axis is given by equating coefficients of y2$y^2$ to zero.

x2−a2=0x=±a$$\begin{array}{cc}{x}^2-a^2& =0\\ x& =\pm a\end{array}$$

We expect 4$4$ asymptotes, let y=mx+c$y=mx+c$ be the equation of the rest, Putting x=1$x=1$ and y=m$y=m$,

ϕ4(m)=1−2m+m2ϕ′4(m)=2m−2ϕ″4(m)=2ϕ3(m)=0ϕ2(m)=−a2−a2m2$$\begin{array}{cc}{\varphi }_4\left(m\right)& =1-2m+m^2\\ {{\varphi }^{\prime }}_4\left(m\right)& =2m-2\\ {{\varphi }^{\prime \prime }}_4\left(m\right)& =2\\ {\varphi }_3\left(m\right)& =0\\ {\varphi }_2\left(m\right)& =-a^2-a^2{m}^2\end{array}$$

The slope of the asymptotes can be found by,

ϕ4(m)=01−2m+m2=0(m−1)(m−1)=0m=1,1$$\begin{array}{cc}{\varphi }_4\left(m\right)& =0\\ 1-2m+m^2& =0\\ (m-1)(m-1)& =0\\ m& =1,1\end{array}$$

This is a case of repeated factors, so

c22!ϕ″4(m)+cϕ′3(m)+ϕ2(m)=0c22×2+0−a2−a2m2=0c2−a2−a2=0c=±√2a$$\begin{array}{cc}\frac{c^2}{2!}{{\varphi }^{\prime \prime }}_4\left(m\right)+c{{\varphi }^{\prime }}_3\left(m\right)+{\varphi }_2\left(m\right)& =0\\ \frac{c^2}{2}\times 2+0-a^2-a^2{m}^2& =0\\ c^2-a^2-a^2& =0\\ c& =\pm \sqrt{2}a\end{array}$$

The asymptotes are thus,

x=±ay=x±√2a$$\begin{array}{cc}x& =\pm a\\ y& =x\pm \sqrt{2}a\end{array}$$

---

3. y3+x2y+2xy2−y+1=0$y^3+x^{2}y+2x{y}^2-y+1=0$

There are no asymptotes parallel to y$y$-axis. Degree of equation is 3$3$, so asymptote parallel to x$x$-axis is obtained by equating the coefficients of highest degree term of x$x$ to 0$0$.

y=0$$\begin{array}{cc}y& =0\end{array}$$

The equation is of form F3+F1=0$F_3+F_1=0$.

y3+x2y+2xy2⏟F3+(−y+1)⏟F1=0$$\begin{array}{c}\underset{F_3}{\underset{}{y^3+x^{2}y+2x{y}^2}}+\underset{F_1}{\underset{}{(-y+1)}}=0\end{array}$$

By inspection method we can obtain asymptotes by equating F3=0$F_3=0$. But we have to make sure that no two linear factors of F3$F_3$ are coincident or differ by constant.

The linear factors of F3$F_3$ are

F3=y3+x2y+2xy2=y2(x+y)+xy(x+y)=y(x+y)(x+y)$$\begin{array}{cc}{F}_3& =y^3+x^{2}y+2x{y}^2\\ & =y^2(x+y)+xy(x+y)\\ & =y(x+y)(x+y)\end{array}$$

Two linear factors are repeated which violates the method of inspection. So we cannot take this approach.

Let y=mx+c$y=mx+c$ be the equation of rest of the asymptotes. Putting x=1$x=1$ and y=m$y=m$,

ϕ3(m)=m3+m+2m2ϕ′3(m)=3m2+1+4mϕ″3(m)=6m+4ϕ2(m)=0ϕ1(m)=−m$$\begin{array}{cc}{\varphi }_3\left(m\right)& =m^3+m+2m^2\\ {{\varphi }^{\prime }}_3\left(m\right)& =3m^2+1+4m\\ {{\varphi }^{\prime \prime }}_3\left(m\right)& =6m+4\\ {\varphi }_2\left(m\right)& =0\\ {\varphi }_1\left(m\right)& =-m\end{array}$$

The slopes of the asymptotes are,

ϕ3(m)=0m3+m+2m2=0m(m2+2m+1)=0m(m+1)(m+1)=0m=0,−1,−1$$\begin{array}{cc}{\varphi }_3\left(m\right)& =0\\ m^3+m+2m^2& =0\\ m(m^2+2m+1)& =0\\ m(m+1)(m+1)& =0\\ m& =0,-1,-1\end{array}$$

When m=0$m=0$, c=−ϕ2(m)ϕ′3(m)=0$c=-\frac{{\varphi }_2\left(m\right)}{{{\varphi }^{\prime }}_3\left(m\right)}=0$. This asymptote is already found. See above.

m=−1$m=-1$ is repeated. So

c22!ϕ″3(m)+cϕ′2(m)+ϕ1(m)=0c22(6m+4)+0−m=0c2=1c=±1$$\begin{array}{cc}\frac{c^2}{2!}{{\varphi }^{\prime \prime }}_3\left(m\right)+c{{\varphi }^{\prime }}_2\left(m\right)+{\varphi }_1\left(m\right)=0& \\ \frac{c^2}{2}(6m+4)+0-m& =0\\ c^2& =1\\ c& =\pm 1\end{array}$$

Equation of the asymptotes are thus,

y=0y+x=±1$$\begin{array}{cc}y& =0\\ y+x& =\pm 1\end{array}$$

---

4. y3−xy2−x2y+x3+x2−y2=1$y^3-x{y}^2-x^{2}y+x^3+x^2-y^2=1$

There are no asymptotes parallel to x$x$-axis and y$y$-axis because coefficients of x3$x^3$ and y3$y^3$ are constants and the degree of equation is 3$3$.

For finding oblique asymptotes in the form y=mx+c$y=mx+c$, put x=1$x=1$ and y=m$y=m$,

ϕ3(m)=m3−m2−m+1ϕ′3(m)=3m2−2m−1ϕ″3(m)=6m−2ϕ2(m)=1−m2ϕ′2(m)=−2mϕ1(m)=0$$\begin{array}{cc}{\varphi }_3\left(m\right)& =m^3-m^2-m+1\\ {{\varphi }^{\prime }}_3\left(m\right)& =3m^2-2m-1\\ {{\varphi }^{\prime \prime }}_3\left(m\right)& =6m-2\\ {\varphi }_2\left(m\right)& =1-m^2\\ {{\varphi }^{\prime }}_2\left(m\right)& =-2m\\ {\varphi }_1\left(m\right)& =0\end{array}$$

The slope of the asymptotes are,

ϕ3(m)=0m3−m2−m+1=0m2(m−1)−1(m−1)=0(m+1)(m−1)(m−1)=0m=1,1,−1$$\begin{array}{cc}{\varphi }_3\left(m\right)& =0\\ m^3-m^2-m+1& =0\\ m^2(m-1)-1(m-1)& =0\\ (m+1)(m-1)(m-1)& =0\\ m& =1,1,-1\end{array}$$

For m=−1$m=-1$,

c=−ϕ2(m)ϕ′3(m)=−1−14=0$$\begin{array}{cc}c& =-\frac{{\varphi }_2\left(m\right)}{{{\varphi }^{\prime }}_3\left(m\right)}\\ & =-\frac{1-1}{4}\\ & =0\end{array}$$

For m=1$m=1$, which is the repeated value of m$m$, for finding c$c$, we have,

c22!ϕ″3(m)+cϕ′2(m)+ϕ1(m)=0c22(6m−2)−2mc+0=0c2×2−2c=0c2−c=0c(c−1)=0c=0,1$$\begin{array}{cc}\frac{c^2}{2!}{{\varphi }^{\prime \prime }}_3\left(m\right)+c{{\varphi }^{\prime }}_2\left(m\right)+{\varphi }_1\left(m\right)=0& \\ \frac{c^2}{2}(6m-2)-2mc+0& =0\\ c^2\times 2-2c& =0\\ c^2-c& =0\\ c(c-1)& =0\\ c& =0,1\end{array}$$

The asymptotes are thus,

y+x=0y=xy=x+1$$\begin{array}{cc}y+x& =0\\ y& =x\\ y& =x+1\end{array}$$

---

22. x3−2x2y+xy2+x2−xy+2=0$x^3-2x^{2}y+x{y}^2+x^2-xy+2=0$

The degree of equation is 3$3$. The equation does not have asymptote parallel to x$x$-axis. The equation does not have y3$y^3$, so the asymptote parallel to y$y$-axis is,

x=0$$\begin{array}{cc}x& =0\end{array}$$

Let y=mx+c$y=mx+c$ be the equation of asymptotes. Putting x=1$x=1$ and y=m$y=m$, we get

ϕ3(m)=1−2m+m2ϕ′3(m)=2m−2ϕ″3(m)=2ϕ2(m)=1−mϕ′2(m)=−1ϕ1(m)=0$$\begin{array}{cc}{\varphi }_3\left(m\right)& =1-2m+m^2\\ {{\varphi }^{\prime }}_3\left(m\right)& =2m-2\\ {{\varphi }^{\prime \prime }}_3\left(m\right)& =2\\ {\varphi }_2\left(m\right)& =1-m\\ {{\varphi }^{\prime }}_2\left(m\right)& =-1\\ {\varphi }_1\left(m\right)& =0\end{array}$$

The slope of asymptotes are given by,

ϕ3(m)=01−2m+m2=0(m−1)(m−1)=0m=1,1$$\begin{array}{cc}{\varphi }_3\left(m\right)& =0\\ 1-2m+m^2& =0\\ (m-1)(m-1)& =0\\ m& =1,1\end{array}$$

This is a case of two repeated roots i.e. two values of m$m$ are same, so

c22!ϕ″3(m)+cϕ′2(m)+ϕ1(m)=0c22×2−c+0=0c2−c=0c(c−1)=0c=0,1$$\begin{array}{cc}\frac{c^2}{2!}{{\varphi }^{\prime \prime }}_3\left(m\right)+c{{\varphi }^{\prime }}_2\left(m\right)+{\varphi }_1\left(m\right)=0& \\ \frac{c^2}{2}\times 2-c+0& =0\\ c^2-c& =0\\ c(c-1)& =0\\ c& =0,1\end{array}$$

The three asymptotes are thus,

x=0y=xy=x+1$$\begin{array}{cc}x& =0\\ y& =x\\ y& =x+1\end{array}$$

---

6. x3−2y3+2x2y−xy2+xy−y2+1=0$x^3-2y^3+2x^{2}y-x{y}^2+xy-y^2+1=0$ [TU 2062]

There are no asymptotes parallel to x$x$-axis and y$y$-axis because the degress is 3$3$ and coefficients of x3$x^3$ and y3$y^3$ are constants.

Let x=1$x=1$ and y=m$y=m$,

ϕ3(m)=1−2m3+2m−m2ϕ′3(m)=−6m2+2−2m$$\begin{array}{cc}{\varphi }_3\left(m\right)& =1-2m^3+2m-m^2\\ {{\varphi }^{\prime }}_3\left(m\right)& =-6m^2+2-2m\end{array}$$

The slope of the asymptotes are given by,

ϕ3(m)=01−2m3+2m−m2=0−1(m2−1)−2m(m2−1)=0(m2−1)(−1−2m)=0(m+1)(m−1)(2m+1)=0m=1,−1,−12$$\begin{array}{cc}{\varphi }_3\left(m\right)& =0\\ 1-2m^3+2m-m^2& =0\\ -1(m^2-1)-2m(m^2-1)& =0\\ (m^2-1)(-1-2m)& =0\\ (m+1)(m-1)(2m+1)& =0\\ m& =1,-1,-\frac{1}{2}\end{array}$$

For c$c$,

c=−ϕ2(m)ϕ′3(m)=−m(1−m)−6m2−2m+2c=m(1−m)6m2+2m−2$$\begin{array}{cc}c& =-\frac{{\varphi }_2\left(m\right)}{{{\varphi }^{\prime }}_3\left(m\right)}\\ & =-\frac{m(1-m)}{-6m^2-2m+2}\\ c& =\frac{m(1-m)}{6m^2+2m-2}\end{array}$$

So,

m$m$	c$c$
1$1$	0$0$
−1$-1$	−1$-1$
−12$-\frac{1}{2}$	12$\frac{1}{2}$

The asymptotes are thus,

• y=x$y=x$
• y+x+1=0$y+x+1=0$
• x+2y=1$x+2y=1$

---

7. (x2−y2)(x+2y+1)+x+y+1=0$(x^2-y^2)(x+2y+1)+x+y+1=0$

The equation can be written as (x+y)(x−y)(x+2y+1)+x+y+1=0$(x+y)(x-y)(x+2y+1)+x+y+1=0$.

The equation has no asymptotes parallel to x$x$-axis. The coefficient of y3$y^3$ is constant, so no asymptotes parallel to x$x$-axis.

The equation is of form F3(x,y)+F1(x,y)=0$F_3(x,y)+F_1(x,y)=0$.

(x+y)(x−y)(x+2y+1)⏟F3+x+y+1⏟F1=0$$\begin{array}{cc}\underset{F_3}{\underset{}{(x+y)(x-y)(x+2y+1)}}+\underset{F_1}{\underset{}{x+y+1}}& =0\end{array}$$

F3(x,y)$F_3(x,y)$ has degree 3$3$ and is product of three different non-repeating linear factors. By method of inspection, thus the asymptotes are obtained by equating F3(x,y)=0$F_3(x,y)=0$,

x+y=0x−y=0x+2y+1=0$$\begin{array}{cc}x+y& =0\\ x-y& =0\\ x+2y+1& =0\end{array}$$

---

8. x(x−y)2−3(x2−y2)+8y=0$x(x-y{)}^2-3(x^2-y^2)+8y=0$ [TU 2060]

Coefficient of x3$x^3$ is constant, no asymptotes parallel to x$x$-axis.

The degree of equation is 3$3$. There is no y3$y^3$, so asymptotes parallel to y$y$-axis is obtained by equating the coefficients of highest degree terms to zero.

x+3=0$$\begin{array}{cc}x+3& =0\end{array}$$

The equation can be written as x(x−y)2−3(x−y)(x+y)+8y=0$x(x-y{)}^2-3(x-y\left)\right(x+y)+8y=0$ which is of form

(y−m1x)2Fn−2+(y−m1x)Gn−2+Pn−2=0$(y-m_{1}x{)}^2{F}_{n-2}+(y-m_{1}x)G_{n-2}+P_{n-2}=0$.

Dividing the equation both sides by, x$x$,

(x−y)2−3(x−y)(x+y)1x+8yx=0$$\begin{array}{cc}(x-y{)}^2-3(x-y\left)\right(x+y)\frac{1}{x}+8\frac{y}{x}& =0\end{array}$$

The two asymptotes parallel to x−y$x-y$ are,

(x−y)2−3(x−y)limx→∞yx→1(x+y)1x+limx→∞yx→18yx=0(x−y)2−3(x−y)limx→∞yx→1{1+yx}+8=0(x−y)2−3(x−y)×2+8=0(x−y)2−6(x−y)+8=0$$\begin{array}{cc}(x-y{)}^2-3(x-y\left)\underset{\begin{array}{c}x\to \infty \\ \frac{y}{x}\to 1\end{array}}{lim}\right(x+y)\frac{1}{x}+\underset{\begin{array}{c}x\to \infty \\ \frac{y}{x}\to 1\end{array}}{lim}8\frac{y}{x}& =0\\ (x-y{)}^2-3(x-y\left)\underset{\begin{array}{c}x\to \infty \\ \frac{y}{x}\to 1\end{array}}{lim}\right\{1+\frac{y}{x}\}+8& =0\\ (x-y{)}^2-3(x-y)\times 2+8& =0\\ (x-y{)}^2-6(x-y)+8& =0\end{array}$$

Solving for x−y$x-y$,

x−y=6±√36−322x−y=2,4$$\begin{array}{cc}x-y& =\frac{6\pm \sqrt{36-32}}{2}\\ x-y& =2,4\end{array}$$

So the asymptotes parallel to x−y$x-y$ are

x−y=2x−y=4$$\begin{array}{cc}x-y& =2\\ x-y& =4\end{array}$$

Thus, the given equation has maximum of three asymptotes, all has been found.

---

9. x3+3x2y−4y3−x+y+3=0$x^3+3x^{2}y-4y^3-x+y+3=0$ [TU 2054, 2055]

There are no asymptotes parallel to x$x$-axis and y$y$-axis.

Lets put x=1$x=1$ and y=m$y=m$. Then,

ϕ3(m)=1+3m−4m3ϕ′3(m)=−12m2+3ϕ″3(m)=−24mϕ2(m)=0ϕ1(m)=m−1$$\begin{array}{cc}{\varphi }_3\left(m\right)& =1+3m-4m^3\\ {{\varphi }^{\prime }}_3\left(m\right)& =-12m^2+3\\ {{\varphi }^{\prime \prime }}_3\left(m\right)& =-24m\\ {\varphi }_2\left(m\right)& =0\\ {\varphi }_1\left(m\right)& =m-1\end{array}$$

The slope of the asymptotes are given by

ϕ3(m)=01+3m−4m3=0(1−m)(4m2+4m+1)=0(1−m)(2m+1)2=0m=1,−12,−12$$\begin{array}{cc}{\varphi }_3\left(m\right)& =0\\ 1+3m-4m^3& =0\\ (1-m)(4m^2+4m+1)& =0\\ (1-m)(2m+1{)}^2& =0\\ m& =1,-\frac{1}{2},-\frac{1}{2}\end{array}$$

For m=1$m=1$,

c=−ϕ2(m)ϕ′3(m)=0$$\begin{array}{cc}c& =-\frac{{\varphi }_2\left(m\right)}{{{\varphi }^{\prime }}_3\left(m\right)}\\ & =0\end{array}$$

So, for m=1$m=1$, y=x$y=x$ is an asymptote.

Now two m$m$ values are same i.e −12$-\frac{1}{2}$. So to find c$c$,

c22!ϕ″3(m)+cϕ′2(m)+ϕ1(m)=0c22!(−24m)+0+m−1=0$$\begin{array}{cc}\frac{c^2}{2!}{{\varphi }^{\prime \prime }}_3\left(m\right)+c{{\varphi }^{\prime }}_2\left(m\right)+{\varphi }_1\left(m\right)& =0\\ \frac{c^2}{2!}(-24m)+0+m-1& =0\end{array}$$

Putting c=−12$c=-\frac{1}{2}$,

−12c2×(−12)−12−1=06c2−32=0c2=14c=±12$$\begin{array}{cc}-12c^2\times (-\frac{1}{2})-\frac{1}{2}-1& =0\\ 6c^2-\frac{3}{2}& =0\\ c^2& =\frac{1}{4}\\ c& =\pm \frac{1}{2}\end{array}$$

So y=−12x±12$y=-\frac{1}{2}x\pm \frac{1}{2}$ are the asymptotes corresponding to m=−12$m=-\frac{1}{2}$. Thus all three asymptotes of the equation are,

x−y=0x+2y+1=0x+2y−1=0$$\begin{array}{cc}x-y& =0\\ x+2y+1& =0\\ x+2y-1& =0\end{array}$$

---

24. (x−1)(x−2)(x+y)+x2+x+1=0$(x-1)(x-2)(x+y)+x^2+x+1=0$ [TU 2059]

The degree of equation is 3. There is no y3$y^3$, so asymptotes parallel to y$y$-axis is obtained by equating the coefficients of highest degree term of y$y$, i.e.

(x−1)(x−2)=0x−1=0,x−2=0$$\begin{array}{ccc}(x-1)(x-2)& =0& \\ x-1& =0,x-2& =0\end{array}$$

The coefficient of x3$x^3$ is constant. So no asymptotes parallel to x$x$-axis.

The equation is of form (y−m1x)Fn−1+Pn−1=0$(y-m_{1}x)F_{n-1}+P_{n-1}=0$.

(x+y)(x−1)(x−2)⏟F3−1+x2+x+1⏟P3−1=0$$\begin{array}{cc}(x+y)\underset{F_{3-1}}{\underset{}{(x-1)(x-2)}}+\underset{P_{3-1}}{\underset{}{x^2+x+1}}& =0\end{array}$$

So the asymptote parallel to x+y$x+y$ is obtained by,

x+y+limx→∞yx→−1x2+x+1(x−1)(x−2)=0x+y+limx→∞yx→−11+1x+1x2(1−1x)(1−2x)=0x+y+1=0$$\begin{array}{cc}x+y+\underset{\begin{array}{c}x\to \infty \\ \frac{y}{x}\to -1\end{array}}{lim}\frac{x^2+x+1}{(x-1)(x-2)}& =0\\ x+y+\underset{\begin{array}{c}x\to \infty \\ \frac{y}{x}\to -1\end{array}}{lim}\frac{1+\frac{1}{x}+\frac{1}{x^2}}{(1-\frac{1}{x})(1-\frac{2}{x})}& =0\\ x+y+1& =0\end{array}$$

Thus three asymptotes are,

• x−1=0$x-1=0$
• x−2=0$x-2=0$
• x+y+1=0$x+y+1=0$

---

14.1.2 Question 5 [TU 2059]

Show that the asymptotes of the curve x2y2=a2(x2+y2)$x^2{y}^2=a^2(x^2+y^2)$ form a square of side 2a$2a$.

The equation can be written as,

x2y2−a2x2−a2y2=0$$\begin{array}{cc}{x}^2{y}^2-a^2{x}^2-a^2{y}^2& =0\end{array}$$

Degree of equation is 4$4$. No x4$x^4$ and y4$y^4$ terms. So asymptotes parallel to x$x$-axis is obtained by equating the coefficients of highest degree term of x$x$.

y2−a2=0y=±a$$\begin{array}{cc}{y}^2-a^2& =0\\ y& =\pm a\end{array}$$

So asymptotes parallel to y$y$-axis is obtained by equating the coefficients of highest degree term of y$y$.

x2−a2=0x=±a$$\begin{array}{cc}{x}^2-a^2& =0\\ x& =\pm a\end{array}$$

The distance between the asymptotes x=a$x=a$ and x=−a$x=-a$ is 2a$2a$.

Similarly, the distance between the asymptotes y=a$y=a$ and y=−a$y=-a$ is 2a$2a$. Thus, two distances are equal and hence it is a square. See the graph below,

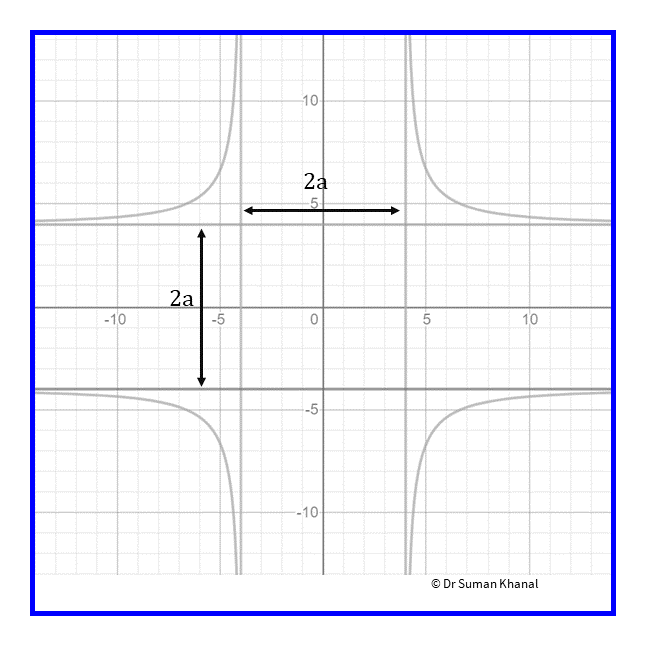
Figure 14.1: Curve and asymptotes of x2y2=a2(x2+y2)$x^2{y}^2=a^2(x^2+y^2)$

---

Trigonometry to remember

• sin(nπ+θ)=(−1)nsinθ$\sin (n\pi +\theta )=(-1{)}^n\sin \theta$
• cos(nπ+θ)=(−1)ncosθ$\cos (n\pi +\theta )=(-1{)}^n\cos \theta$

Function	Domain	Range
sin−1(x)${\sin }^{-1}\left(x\right)$	[−1,1]$[-1,1]$	[−π/2,π/2]$[-\pi /2,\pi /2]$
cos−1(x)${\cos }^{-1}\left(x\right)$	[−1,1]$[-1,1]$	[0,π]$[0,\pi ]$
tan−1(x)${\tan }^{-1}\left(x\right)$	(−∞,∞)$(-\infty ,\infty )$	(−π/2,π/2)$(-\pi /2,\pi /2)$
cot−1(x)${\cot }^{-1}\left(x\right)$	(−∞,∞)$(-\infty ,\infty )$	(0,π)$(0,\pi )$
sec−1(x)${\sec }^{-1}\left(x\right)$	(−∞,−1]∪[1,∞)$(-\infty ,-1]\cup [1,\infty )$	[0,π/2)∪(π/2,π]$[0,\pi /2)\cup (\pi /2,\pi ]$
csc−1(x)${\csc }^{-1}\left(x\right)$	(−∞,−1]∪[1,∞)$(-\infty ,-1]\cup [1,\infty )$	[−π/2,0)∪(0,π/2]$[-\pi /2,0)\cup (0,\pi /2]$

Rules for finding asymptotes of polar curves

• Put u=1r$u=\frac{1}{r}$ and write the equation in form u=F(θ)$u=F\left(\theta \right)$.
• Find θ$\theta$ for which F(θ)=0$F\left(\theta \right)=0$. This value will be θ1${\theta }_1$.
• Find F′(θ1)$F^{\prime }\left({\theta }_1\right)$.
• The equation of the asymptotes are then obtained by plugging by θ1${\theta }_1$ and F′(θ1)$F^{\prime }\left({\theta }_1\right)$ pairs in the equation rsin(θ−θ1)=1F′(θ1)$r\sin (\theta -{\theta }_1)=\frac{1}{F^{\prime }\left({\theta }_1\right)}$.

14.1.3 Question 6

Find the asymptotes of the curves

1. 2r2=tan2θ$2r^2=\tan 2\theta$

Putting u=1r$u=\frac{1}{r}$, then

u2=2cot2θu=√2cot2θ=F(θ)$$\begin{array}{cc}{u}^2& =2\cot 2\theta \\ u& =\sqrt{2\cot 2\theta }=F\left(\theta \right)\end{array}$$

When r→∞$r\to \infty$, u→0$u\to 0$, or

√2cot2θ=0cot2θ=02θ=cot−102θ=π2, not −π/2 because the domainof arccot function is closed interval (0,π)θ=π4$$\begin{array}{cc}\sqrt{2\cot 2\theta }& =0\\ \cot 2\theta & =0\\ 2\theta & ={\cot }^{-1}0\\ 2\theta & =\frac{\pi }{2},\text{not}-\pi /2\text{because the domain}\\ & \text{of arccot function is closed interval}(0,\pi )\\ \theta & =\frac{\pi }{4}\end{array}$$

i.e. when r→∞$r\to \infty$, θ→π/4$\theta \to \pi /4$. So,

θ1=π4$$\begin{array}{cc}{\theta }_1& =\frac{\pi }{4}\end{array}$$

Differentiating F(θ)$F\left(\theta \right)$ w.r.t θ$\theta$,

F′(θ)=dudθ=−4csc22θ√2cot2θF′(θ1=π/4)=−40=∞$$\begin{array}{cc}{F}^{\prime }\left(\theta \right)& =\frac{du}{d\theta }=\frac{-4{\csc }^{2}2\theta }{\sqrt{2\cot 2\theta }}\\ F^{\prime }({\theta }_1=\pi /4)& =\frac{-4}{0}=\infty \end{array}$$

The equation of the asymptote is then given by,

rsin(θ−θ1)=1F′(θ1)rsin(θ−π/4)=1∞rsin(θ−π/4)=0θ−π/4=sin−10θ−π/4=0, domain of arcsin is [−π/2,π/2]θ=π4$$\begin{array}{cc}r\sin (\theta -{\theta }_1)& =\frac{1}{F^{\prime }\left({\theta }_1\right)}\\ r\sin (\theta -\pi /4)& =\frac{1}{\infty }\\ r\sin (\theta -\pi /4)& =0\\ \theta -\pi /4& ={\sin }^{-1}0\\ \theta -\pi /4& =0,\text{domain of arcsin is}[-\pi /2,\pi /2]\\ \theta & =\frac{\pi }{4}\end{array}$$

---

2. rθ=a$r\theta =a$

Put u=1r$u=\frac{1}{r}$.

So u=θa=F(θ)$u=\frac{\theta }{a}=F\left(\theta \right)$.

When r→∞$r\to \infty$, u→0$u\to 0$ or

θa=0θ=0$$\begin{array}{cc}\frac{\theta }{a}& =0\\ \theta & =0\end{array}$$

i.e. when r→∞$r\to \infty$, θ→0$\theta \to 0$. So

θ1=0$$\begin{array}{cc}{\theta }_1& =0\end{array}$$

Also,

F′(θ)=1aF′(θ1=0)=1a$$\begin{array}{cc}{F}^{\prime }\left(\theta \right)& =\frac{1}{a}\\ F^{\prime }({\theta }_1=0)& =\frac{1}{a}\end{array}$$

The equation of the asymptote is thus,

rsin(θ−θ1)=1F′(θ1)rsin(θ−0)=arsinθ=a$$\begin{array}{cc}r\sin (\theta -{\theta }_1)& =\frac{1}{F^{\prime }\left({\theta }_1\right)}\\ r\sin (\theta -0)& =a\\ r\sin \theta & =a\end{array}$$

Second method

Here, the given equation is a hyperbolic spiral. The equation can be written as,

r=aθ, where θ>0$$\begin{array}{cc}r& =\frac{a}{\theta },\text{where}\theta >0\end{array}$$

We know,

x=rcosθx=acosθθ, from above$$\begin{array}{cc}x& =r\cos \theta \\ x& =a\frac{\cos \theta }{\theta },\text{from above}\\ & \end{array}$$

Lets see the behavior of x$x$ when θ→0+$\theta \to 0^{+}$.

x=alimθ→0+cosθθ=+∞$$\begin{array}{cc}x& =a\underset{\theta \to 0^{+}}{lim}\frac{\cos \theta }{\theta }\\ & =+\infty \end{array}$$

Similarly,

y=rsinθy=asinθθ$$\begin{array}{cc}y& =r\sin \theta \\ y& =a\frac{\sin \theta }{\theta }\end{array}$$

Lets see the behavior of y$y$ when θ→0+$\theta \to 0^{+}$.

y=alimθ→0+sinθθy=a×1y=arsinθ=a$$\begin{array}{cc}y& =a\underset{\theta \to 0^{+}}{lim}\frac{\sin \theta }{\theta }\\ y& =a\times 1\\ y& =a\\ r\sin \theta & =a\end{array}$$

Thus, y=a$y=a$ or rsinθ=a$r\sin \theta =a$ is the horizontal asymptote of the given equation.

---

3. rsinθ=a$r\sin \theta =a$

Transforming the equation into cartesian form, the equation can be written as,

y=a$$\begin{array}{cc}y& =a\end{array}$$

This is equation of a straight line. Asymptote in case of straight line does not make sense. No asymptote !

---

4. rθcosθ=acos2θ$r\theta \cos \theta =a\cos 2\theta$

Asymptotes are the lines which touch the curve at infinity.

Putting u=1r$u=\frac{1}{r}$, then

u=θcosθacos2θ=F(θ)$$\begin{array}{cc}u& =\frac{\theta \cos \theta }{a\cos 2\theta }=F\left(\theta \right)\end{array}$$

When r→∞$r\to \infty$, u→0$u\to 0$, or

θcosθacos2θ=0θcosθ=0θ=0,cosθ=0θ=0,θ=cos−10θ=0,θ=π2, and not−π2 because inverse cos function is defined only in the interval [0,π]$$\begin{array}{cc}\frac{\theta \cos \theta }{a\cos 2\theta }& =0\\ \theta \cos \theta & =0\\ \theta & =0,\cos \theta =0\\ \theta & =0,\theta ={\cos }^{-1}0\\ \theta & =0,\theta =\frac{\pi }{2},\text{and not}-\frac{\pi }{2}\\ & \text{because inverse cos function is defined only in the interval}[0,\pi ]\end{array}$$

i.e. when r→∞$r\to \infty$, θ→0,π2$\theta \to 0,\frac{\pi }{2}$. So,

θ1=0,π2$$\begin{array}{cc}{\theta }_1& =0,\frac{\pi }{2}\end{array}$$

Differentiating F(θ)$F\left(\theta \right)$ w.r.t θ$\theta$,

F′(θ)=acos2θddθ(θcosθ)−θcosθddθ(acos2θ)a2cos22θF′(θ)=acos2θ(cosθ−θsinθ)+2aθcosθsin2θa2cos22θ$$\begin{array}{cc}{F}^{\prime }\left(\theta \right)& =\frac{a\cos 2\theta \frac{d}{d\theta }\left(\theta \cos \theta \right)-\theta \cos \theta \frac{d}{d\theta }\left(a\cos 2\theta \right)}{a^2{\cos }^{2}2\theta }\\ F^{\prime }\left(\theta \right)& =\frac{a\cos 2\theta (\cos \theta -\theta \sin \theta )+2a\theta \cos \theta \sin 2\theta }{a^2{\cos }^{2}2\theta }\end{array}$$

θ1${\theta }_1$	F′(θ1)$F^{\prime }\left({\theta }_1\right)$
0$0$	1a$\frac{1}{a}$
π2$\frac{\pi }{2}$	π2a$\frac{\pi }{2a}$

The equation of the asymptote in case of polar curves is given by,

rsin(θ−θ1)=1F′(θ1)

So in our case, asymptotes are,

θ1	Equation of asymptote
0	rsin(θ−0)=11arsinθ=a
π/2	rsin(θ−π2)=2aπ2a+πrcosθ=0